When a single-phase induction motor is supplied from a source, the flux produced will be alternating in nature. Due to transformer action, an e.m.f is induced in the stationary rotor bars and the flux produced will also be alternating. As the stator flux and the rotor flux have the same magnetic axis, they lack to have the relative motion between the two, which in tum leads to failure in rotor rotation. If this rotor is given an initial push in any direction it continues to run. The reason why the rotor rotates in the direction of push is by using “Double Revolving Field Theory“.
Double Revolving Field Theory
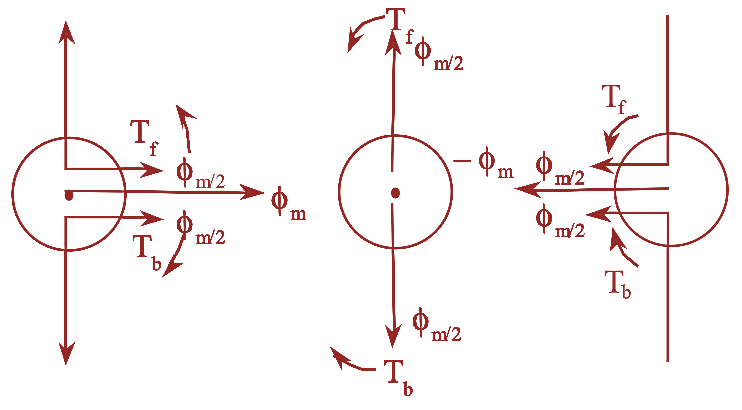
This theory utilizes the Ferrari’s principle which states that “An alternating m.m.f can be resolved into two m.m.fs whose amplitude is half of the original m.m.f and are rotating in opposite directions with equal angular velocity.
Accordingly as the flux produced is alternating in nature (ϕm), they can be resolved into two equal fluxes. Let them be,
1. Forward flux (ϕm/2)
2. Backward flux (ϕm /2).
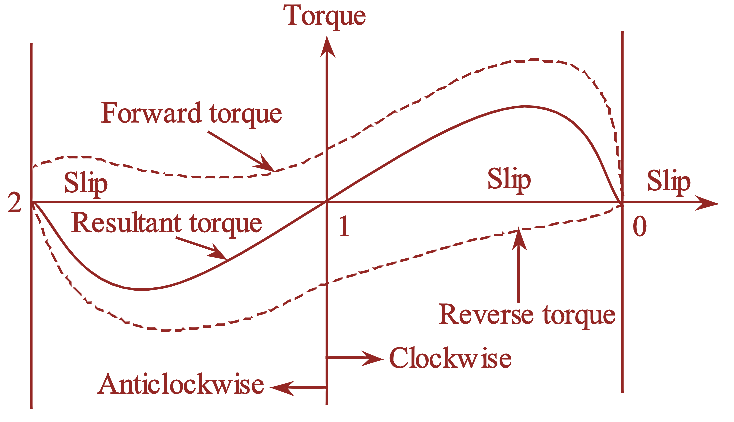
These two fluxes are considered to have equal magnitude revolving at synchronous speed (Ns = 120 f/P) but in opposite directions. During standstill (at slip, s = 1), the two fluxes produce two torques, which are equal and nullify each other at every instant due to which the rotor rejects to move. Thus, it is considered that the 1-ϕ induction motor is not self-starting.
If an initial push is given in any of the directions. let us consider in the direction of forward flux, the forward slip, sf starts reducing from 1 and the backward slip, sb increases above 1 i.e., (2 – s). The current drawn due to backward flux will be higher in magnitude and the power factor will be lesser. This higher current How’s in the direction so as to reduce the main flux, thereby reducing the backward flux. This in tum increases the strength of the forward field so as to keep the sum of two fluxes constant. This strengthened forward field produces higher torque in the same direction keeping the rotor in rotating state continuously. The same is the case when the initial push is given in reverse direction. It continues to run in same direction.