What is Thevenin’s Theorem? Statement, Formula, Steps & Examples

Thevenin’s Theorem is very useful particularly when the current in one branch of a network is to be determined.

Statement of Thevenin’s Theorem

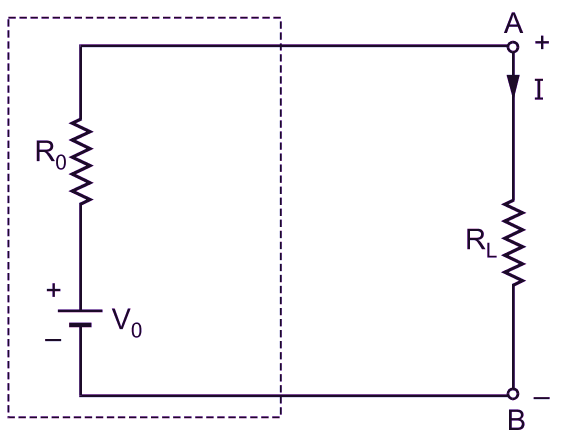
Thevenin's Theorem states that for the purpose of determining the current through a resistor RL (called load resistance) connected across any two terminals A and B of a linear bilateral network with constant resistances, and constant voltage and current sources (Fig. 1 a), the network excluding RL (enclosed by dotted line in Fig. 1 a), can be replaced by a single source of e.m.f. and a series resistor (enclosed by dotted line in Fig. 1 b) where

(i) this e.m.f is equal to the open-circuit voltage, say Vo, between the terminals under consideration (i.e. the voltage across the two terminals A and B with RL removed) and,

(ii) the resistance of the series resistor, say Ro, is equal to the resistance of the network as viewed from the terminals A and B with the load resistance RL removed amid all sources replaced by their internal resistances.

Hence, the current through R_L,

\[\text{I }=\text{ }\frac{{{\text{V}}_{\text{o}}}}{{{\text{R}}_{\text{o}}}+{{\text{R}}_{\text{L}}}}\]

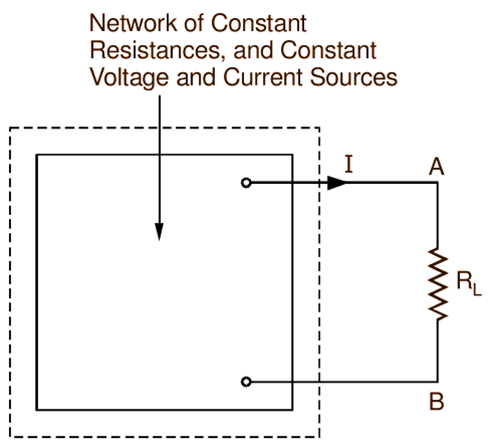
(a)

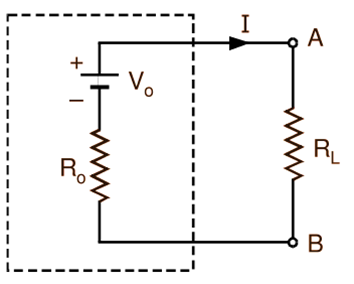
(b)

Fig. 1: General two-terminal network with Thevenin’s equivalent circuit.

Thevenin’s Theorem Formula

Current through the load resistance connected across any two terminals A and B of a linear bilateral network with constant resistances, constant voltage and current sources,

\[\text{I }=\text{ }\frac{{{\text{V}}_{\text{o}}}}{{{\text{R}}_{\text{o}}}+{{\text{R}}_{\text{L}}}}\]

where

R_L = Load resistance connected across the terminals A and B.

V₀ = Thevenin’s equivalent voltage i.e. the open-circuit voltage between the terminals A and B with the load resistance R_L removed.

R₀ = Thevenin’s equivalent resistance i.e. the resistance of the network as viewed from the terminals A and B with the load resistance R_L removed and all sources replaced by their internal resistances.

Explanation of Thevenin’s Theorem

To understand the application of the above theorem more clearly, let us consider the network shown in Fig. 2 (a). It consists of resistors R₁, R₂, and R_L, and a battery having an e.m.f. E and an internal resistance r. If it is required to find the current through a load resistance R_L using Thevenin’s theorem, the procedural steps are as follows:

(i) Remove the load resistance R_L from the terminals A and B and find the open-circuit voltage V₀ between these terminals. Referring to Fig. 2 (b), it is obvious that

\[\text{Current through }{{\text{R}}_{\text{1}}}=\text{ }\frac{\text{E}}{{{\text{R}}_{\text{1}}}+\text{r}}\]

and

\[\text{p}\text{.d across }{{\text{R}}_{\text{1}}}=\text{ }\frac{\text{E }{{\text{R}}_{\text{1}}}}{{{\text{R}}_{\text{1}}}+\text{r}}\]

Since, no current is flowing through R₂, open-circuit voltage across the terminals A and B is

\[{{\text{V}}_{\text{o}}}=\text{ }\frac{\text{E }{{\text{R}}_{\text{1}}}}{{{\text{R}}_{\text{1}}}+\text{r}}\]

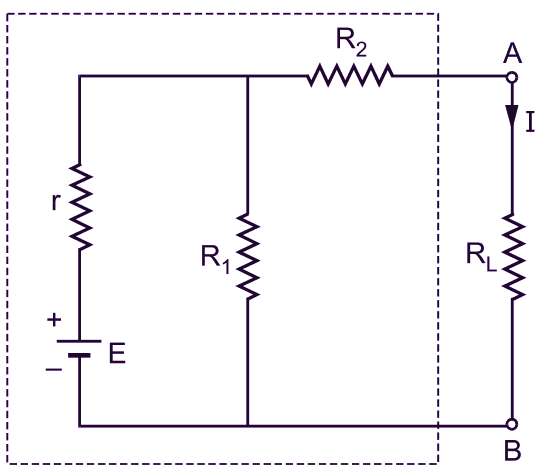
(a)

(b)

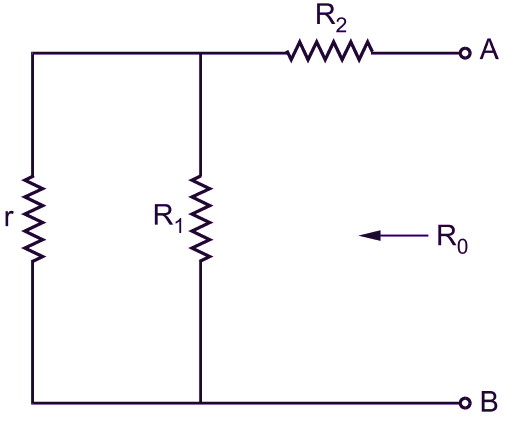
(c)

(d)

Fig. 2: Circuits to illustrate Thevenin’s theorem

(ii) With the load resistance R_L removed and the battery replaced by its internal resistance r as shown in Fig. 2 (c), find the Thevenin’s equivalent resistance R₀ of the network as viewed from the terminals A and B. Obviously,

\[{{\text{R}}_{\text{o}}}=\text{ }{{\text{R}}_{\text{2}}}+\text{ }\frac{\text{r }{{\text{R}}_{\text{1}}}}{{{\text{R}}_{\text{1}}}+\text{r}}\]

(iii) Replace the entire network enclosed by the dotted line in Fig. 2 (a) by the Thevenin’s equivalent circuit consisting of single voltage source with e.m.f. equal to V₀ and internal resistance equal to R₀ as illustrated in Fig. 2 (d).

(iv) Finally, from the simple circuit of Fig. 2 (d), calculate the current I flowing through the load resistance R_L using the expression

\[\text{I }=\text{ }\frac{{{\text{V}}_{\text{o}}}}{{{\text{R}}_{\text{1}}}+{{\text{R}}_{\text{L}}}}\]

Examples of Thevenin’s Theorem

Example 1 : Using Thevenin’s theorem, calculate the current in the 15 Ω resistor in the network shown in Fig. 3.

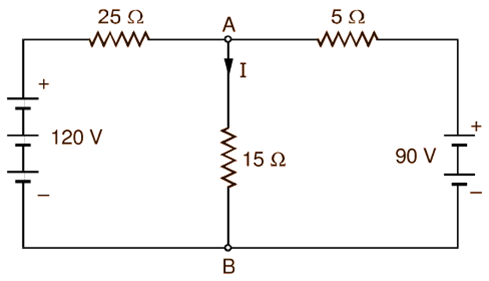
Fig. 4.

Solution 1: For convenience, the network under consideration is redrawn in Fig. 3. Open circuiting the branch containing 15 Ω resistor gives the network shown in Fig. 4 (a). To calculate the open-circuit voltage V₀ across the terminals A and B, assume a current I₁ in the direction shown. Applying the Kirchhoff’s voltage law to the outer loop, we get

120 – 25I₁ – 5I₁ – 90 = 0

∴                                           I₁ = 1 A

(a)

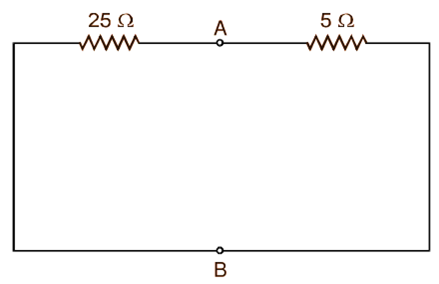
(b)

Fig. 4: (a) Network of Fig. 4 with the 15  resistor removed, (b) Network of Fig. 4 (a) with voltage sources suppressed.

From the voltage law, the open-circuit voltage across the terminals A and B is then

V_o = 120 – 25 × 1 = 95 V

To determine the Thevenin’s equivalent resistance R_o, the voltages of the sources are reduced to zero and the resistance between the terminals A and B is calculated. Fig. 4 (b) shows the network after the suppression of the voltage sources. The 25 Ω and 5 Ω resistances being in parallel between the terminals A and B,

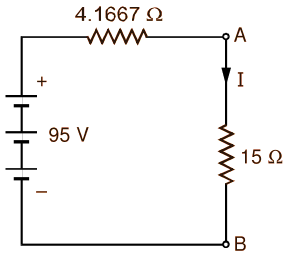
Fig. 5 : Thevenin’s equivalent of the circuit of Fig. 3.

The Thevenin’s equivalent circuit for the network of Fig. 4 (a) between the terminals A and B with 15 Ω resistance added is then as shown in Fig. 5. Hence, current through 15 Ω resistance is

\[\text{I }=\text{ }\frac{{{\text{V}}_{\text{o}}}}{{{\text{R}}_{\text{1}}}+{{\text{R}}_{\text{L}}}}\text{ }=\text{ }\frac{\text{95}}{\text{401667}+\text{15}}\]

\[=\text{ 4}\text{.9565 A}\]